Harbor Sedimentation

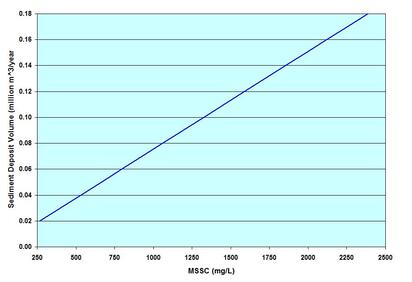A harbor is a water basin of tranquil or tolerable wave and current climate, and of sufficient water depth in which a maritime or inland vessel (let us use this general term, but when Dead Weight Tonnage or DWT ≥ 500, a vessel is known as a ship) can operate safely. Maritime harbors are selected from the deep shoreline areas sheltered naturally, or are created artificially (see Flood Barrier Systems). The artificial harbors are configured and engineered within an ambient water body at the shoreline by dredging and installing suitable structures (see Breakwater). The purpose in each case is to locate a maritime port or marina within it (see Ship Motion and Mooring Restraints; and Propwash). For the convenience of design and operation, a harbor is classified and distinguished as deep-draft (water depth > 15 ft or 4.6 m), and shallow-draft or small-craft (water depth < 15 ft or 4.6 m). Many artificial harbors have one inlet to allow influx and efflux of water and sediment into the basin (a semi-enclosed basin that allows restricted/controlled entry and exit of matter and energy, see Upslope Events and Downslope Processes); and entry and exit of vessels. The layout of the structure – and the location, width and depth of the approach channel as well as of the harbor itself are designed by addressing such constraints as – ambient wave, current and sediment climates, and the largest allowable vessel designed to call at the port.
.  .  .
In this piece, let us attempt to discuss and understand the sedimentation rates of harbors in simple terms. Sediment transport dynamics and sedimentation pose a complicated problem. But ballpark estimates and numbers are always handy and useful to conceive and study the feasibility of a project. To that end, some methods and pieces of data are selected and blended in this piece. The purpose is to demonstrate the usefulness of some simple analytical models that can be used as a handy tool to picture a high-level impression of possible harbor sedimentation. The magnitude of sedimentation problem can be appreciated if one considers worldwide dredging operations. Maintaining enough water depth within the harbor and keeping the approach channels navigable – are some of the requirements that let flourishing of huge dredging industries. These two major demands, together with the erosion prevention and value-adding beach nourishment works, and others – have yielded the global dredging industry to an annual turnover of some $5.6 billion. I will try to come back to discussing different interesting aspects of dredging at a later time.
Among others, this piece is primarily based on: RB Krone 1962; Delft Hydraulics publications (E Allersma 1982; WD Eysink and H Vermass 1983 and WD Eysink 1989); R Soulsby 1997; USACE 2002 EM 1110-2-1100 (Part III) and 2006 EM 1110-2-1110 (Part II); I Smith 2006; and my own works on fine sediments and sedimentation (Fluid Mud 2008; and Settling Velocity of Natural Sediments 2004) published in the Journal of Hydraulic Engineering, and Journal of Waterway, Port, Coastal and Ocean Engineering, respectively; Seabed Roughness; and two papers presented at the International Symposiums on Coastal Ocean Space Utilization: COSU 1995 and COSU 1993, and my paper at the 24th International Conference on Coastal Engineering, Kobe, Japan, ICCE 1994. The Hydraulics of Sediment Transport and Resistance to Flow posted earlier laid out some fundamentals of sediment behavior and transport.
.  .  .
1. Configuring Harbor Layout and Tidal Hystereisis
Configuring the layout of a harbor entrance needs careful optimization exercises and analyses – on the one hand, it has to provide effective diffractive energy dissipation of incoming waves – on the other, it has to minimize the formation and strength of current eddies at the entrance, and sedimentation inside the basin. Filling and emptying tidal currents at a harbor entrance are usually an order of magnitude less than the ambient tidal current. Their magnitudes depend on the size of the basin and entrance. Eddies – more vigorous during changing current directions – are undesirable for at least two primary reasons. The first is to minimize navigation hazards – to vessels entering and leaving the port. The second is to minimize scour and formation of sandy bars. Exercises to engineer a detailed and optimal layout include physical scale modeling and/or numerical modeling. Such exercises, especially the efforts of numerical modeling (see Water Modeling) are becoming increasingly common not only for optimizing harbor entrance layout, but also for visualizing the sediment morphodynamics, sedimentation and other aspects of harbor hydraulics (e.g. Ports 2013 paper).
Before moving on, let us have some words on tidal action. It is assumed that actions attributed to short-waves (see Ocean Waves and Linear Waves) and vessel generated wake-waves are minimal – a valid assumption for all harbors. The main concern of harbor sedimentation processes is the behavior of Suspended Sediment Concentration (SSC) that has a positive gradient from low at top to high at bottom of the water column. As flood and ebb currents reach threshold for erosion and resuspension during a tidal period – sediments are picked up from the seabed and are transported (coarser fraction close to the bed; fines up in the water column) back and forth by the current. Similar but opposite episodes happen, as flood and ebb currents slow down to reach deposition threshold. Suspended sediments have the opportunity to settle down during such slack water periods (SWP) – with more chances for sediments close to the bed than those up in the water column. However, there is often a settlement lag or incoherence between the slack water and actual deposition (see more in my 1990 Elsevier paper).
.  .  .
2. Harbor Sedimentation Problems
Let us now dive down into the core issue of this piece. A harbor faces at least two types of major sedimentation problems. The first is the formation of localized shoals or sandbars at and around the entrance due to the scouring actions of eddies, and the sudden drop in flow velocities. These shoals mostly of sandy materials are often attached to the shoreline as a side bar or develop as middle bar(s). They mostly develop when the harbor entrance is located on littoral shores (see Managing Coastal Inlets) – and are usually termed as flood-tidal and ebb-tidal deltas (see Coastal River Delta and Managing Coastal Inlets). The dynamics of such sandy shoals, bars or deltas can best be discerned from the piece on The Hydraulics of Sediment Transport.
The focus of this piece is on the second type of sedimentation problem. It is the sedimentation of fine sediments within the harbor basin. This sedimentation (a phenomenon of suspended sediments having very low settling velocities) is somewhat uniform due to the relatively weak circulation within the harbor basin – but is often less in areas of relatively high currents than in remote areas of stagnant water. It is highly problematic when a harbor is located within Turbidity Maximum (TM) zone (1990 Elsevier paper). The presence of TM in the tide-dominated east shore channels and waterways of the Ganges-Brahmaputra-Meghna (GBM) River mouth has shown very high siltation rates of fine sediments (1997 Taylor & Francis paper). Observations at the mouth of Karnafuli River estuary showed a positive correlation between the Surface Suspended Sediment Concentration (SSSC) and tidal range (TR) – indicating that the resuspension actions of tidal currents are directly related to tidal range. This correlation ends up yielding an exponential relation between SSSC and TR (ICCE 1994). The fitted relation shows, for example, that at mean neap-tidal TR = 1.7 m, SSSC = 154 mg/L; and at mean spring tidal TR = 3.8 m, SSSC = 1912 mg/L.
The gradual but slow filling up of the basin is highly dependent on the concentration of sediment suspended (in textures of fine sand, silt and clay) of influx water. For the convenience of discussion, let us spilt the piece in two: (1) the first is on sedimentation of granular (silt-sized particles) materials; and (2) the second is on sedimentation of silty/clayey materials that are affected by aggregation and flocculation. The provided estimates represent only a high-level first-order magnitude – afforded by some approximations and assumptions. And to be simple yet realistic of a deep-draft harbor, let us use most of the same inlet/basin/tide parameters (inlet depth 15 m; harbor depth 10 m; semi-diurnal tidal period 12.42 hours; and tidal amplitude 1 m) as described in Managing Coastal Inlets – for a large harbor area of 1 million square meter; and an inlet length and width of 100 m and 300 m, respectively. A tide of this amplitude at 15 m water depth, causes a passing peak depth-averaged current of about 0.81 m/s in front of the harbor. A rough estimate shows that for a harbor of this size at this tidal condition – the turnover time (the time required to tidal flushing out 63% of the harbor water volume) is about 3.2 days.
.  .  .
3. Sedimentation of Suspended Particulates
Let us attempt to make a simple first-order estimate for a single inlet harbor that has no freshwater drainage into it. It is assumed the system is well-mixed vertically without any density stratification. In such harbors, the volumetric sedimentation rates can simply be expressed as a linear function of sediment influx, and a factor attributed to the fraction of sediments that actually settles into the basin. It is also a linear and reciprocal function of dry bulk density of the suspended deposits. One can also assign a calibration factor to account for uncertainty of assumptions, approximations, the approach itself, and for the spatial and temporal distributions of sedimentation.
The first, the sediment influx is a function of SSC that are transported by the total volume of water exchanged during the tidal cycle. This volume, in turn depends on the tidal prism forced into the harbor, and the horizontal exchange of water induced by passing current at the inlet entrance (a function of passing current, inlet width and depth). The Volumetric Suspended Sediment Concentration (VSSC) is reciprocal to the porosity of water-sediment mixture (the ratio of void volume filled with water to the total volume). When VSSC is multiplied by the density of sediment particulates, the Mass Suspended Sediment Concentration (MSSC) is obtained.
The second, the settling factor is a function of (1) particle settling velocity, (2) average basin water depth, (3) threshold velocity for sediment settlement, and (4) the window of time or SWP during a tidal cycle when settling occurs. In this simple example, one silt-sized particulate is considered – the median diameter of which is assumed as 1/32 mm or 0.03125 mm (corresponding to the demarcation defining the coarse and medium silts) – with no sand content. The settling velocity of this fine silty material is 0.084 cm/s (according to Rubey 1933; see 2004).
In the density of sediment-water mixture, let us assume the sediment granular density as 2650 kg/m^3 (typical of silty materials of quartz mineral origin) – and the water density as 1025 kg/m^3 (typical of most coastal water; note this density may be high for the some of the considered cases, but the errors caused by using different water densities are very negligible, see Coastal Water). For all-silt suspended sediment, the dry bulk density of the deposit – is a function of these two parameters, as well as of packing.
Having laid out these fundamental parameters, let us attempt to make some estimates. The included image shows the annual volume of silt-sized sediment deposits as a function of MSSC. It is applicable for parameters discussed earlier with the assumption that the time window available for settling SWP = 10 min during the tidal cycle. As an example, if an MSSC of 1325 mg/L persists throughout the year, the total annual deposit volume will be about 0.10 million m^3. Such a sedimentation rate is no reflection – however, of the usual differences and variations expected in space (e.g. flowing channel vs sheltered areas) and in time (e.g. spring vs neap tides; and dry vs wet season). Based on actual field conditions, sedimentation volume (which can be discerned from dredged volumes; or from repeated bathymetric surveys) and harbor activities (caused by vessel propwash with consequent erosion, resuspension and deposition of sediments; see Propwash), one can apply a factor to calibrate the estimated sedimentation.
.  .  .
4. Sedimentation of Aggregated Particulates
This part of the piece is based mostly on my published papers: the 1994 ICCE 24th; and the COSU 1993 and 1995 papers. The published works are based on some site-specific information; and are therefore primarily applicable for situations and conditions in which they were derived. But the approach and methodology can be applied elsewhere with some assumptions for cases – of tide-dominated estuaries, bays and waterways dominated by fine seabed sediments. Let us attempt to see some applications of the gained experience in simple terms. They are supplemented by my discussions in 2004 and 2008 publications.
The first approach relies on ICCE 1994 paper and the Fluid Mud 2008 discussion. To be realistic of the annual variability, one needs to consider both spring-neap tidal and seasonal parameters. To that end, the used values are: neap and spring tidal TRs as 1.7 m and 4.0 m, respectively, and the wet and dry season salinities as 2.0 ppt (parts per thousand) and 4.0 ppt, respectively. Another assumption has to be made – it is on SWP during which tidal currents slows down below the sedimentation threshold. Experience tells that neap tidal SWP is longer than the spring tidal SWP; therefore let us assume neap SWP = 15 min and spring SWP = 5 min. Let us also consider the neap and spring tidal SSSC as the initial concentrations required for the relations proposed in 2008. With these assumptions and applied parameters as if they persist throughout the year, the total annual sedimentation volume turns out to be about 0.39 million m^3.
The second approach (COSU 1993 and 1995) is primarily based on the modeled sedimentation rates observed in some areas of the GBM mouth – therefore is highly site specific. They were done in contexts of determining the height of sedimentation at which impoldering (a Dutch term referring to the process of reclaiming and developing the silted coastal shores for rice farming – by closing and isolating the area from saline coastal water flooding by installing earthen dikes and sluices) can be initiated. In this case the used relation giving annual sedimentation height relies on initial water depth, and a coefficient. Let me cite examples for two sites – in one, mainly dominated by tide and muddy seabed; and the other dominated by GBM fresh water flow and sand/silty seabed. The measured SSC at the two sites differ by an order of magnitude – the first is about 4230 mg/L, the second is about 126 mg/L. Note that the first area was very sheltered – which means, water flooding the tidal flat and gullies highly laden with SSC was ideal for settling sediments. If one attempts to extrapolate the estimated deposition to the whole harbor basin – applying the assumed conditions and circumstances, the estimated volumes turns out to be 5.42 million m^3 and 0.23 million m^3, respectively.
In summary, the discussed ballpark estimates varying from 0.10 to 0.39 million m^3 – present a clue on what sedimentation to expect when planning the feasibility of a harbor. One referral case in point (see Ports 2013 paper) is the modeled annual sedimentation volume of 0.025 million m^3 – concentrated mostly in entrance areas of the Salt Ponds Inlet harbor (size, 0.1 million m^2) in Virginia, USA. This was the case of a small harbor of sedimentary environment dominated by fine sands, not adequately sheltered from wave actions. The estimate of 5.42 million m^3 – rather unusually high, is an indication of some very calm and stagnant areas – flooded by very high SSC of flocculated sediments. Such a situation is unlikely to happen in harbor conditions.
.  .  .
Before finishing I like to tell a story the Buddha (624 – 544 BCE) told to a congregation of monks and lay people. He did this in context of the 4th Buddhist precept of Right Speech (see Revisiting the Jataka Morals – 2). Once an angry and hateful person used very harsh and abusive words to the Buddha. Instead of getting provoked, the Buddha calmly listened and told the man to take back his abusive outburst. The man was dumbfounded hearing such an unusual reaction. The Buddha then said: Hold it there. If a person gives a gift to another, and if the second person refuses to accept the gift, to whom the gift belongs? The man replied: it belongs to the first person. The Buddha said: so, my friend you must take back the abusive language you have used, because it belongs to you and I refuse to accept it. Then the Buddha delivered some words of wisdom to the congregation: if someone spits against the sky, the spittle returns back to the spitter. So, be mindful. If you use an abusive or unwholesome speech, it gets back at you.

.  .  .  .  .

- by Dr. Dilip K. Barua, 23 November 2020

---